
In surface area questions, we need to know all three side lengths of the triangle however we only need the base and the height to calculate the area of the triangle.
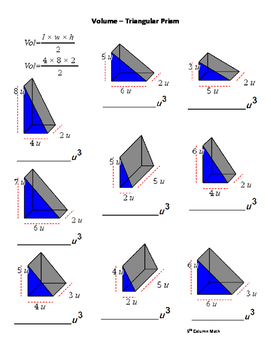
If A is the area of the base triangle and h is the height of the prism then volume of the prism is given by. Formula for the volume of a triangular prism. A triangular prism is a prism that has two congruent parallel triangles as its bases and rectangular lateral faces. Volume and surface area are different things – volume tells us the space within the shape whereas surface area is the total area of the faces. In this lesson we find the volume of a triangular prism. Calculating volume instead of surface area.You can’t have some measurements in cm and some in mīe careful to apply the correct prism related formula to the correct question type. You need to make sure all measurements are in the same units before calculating volume.Į.g. Surface area is measured in units squared (e.g. You should always include units in your answer. Step-by-step guide: Surface area of a triangular prism
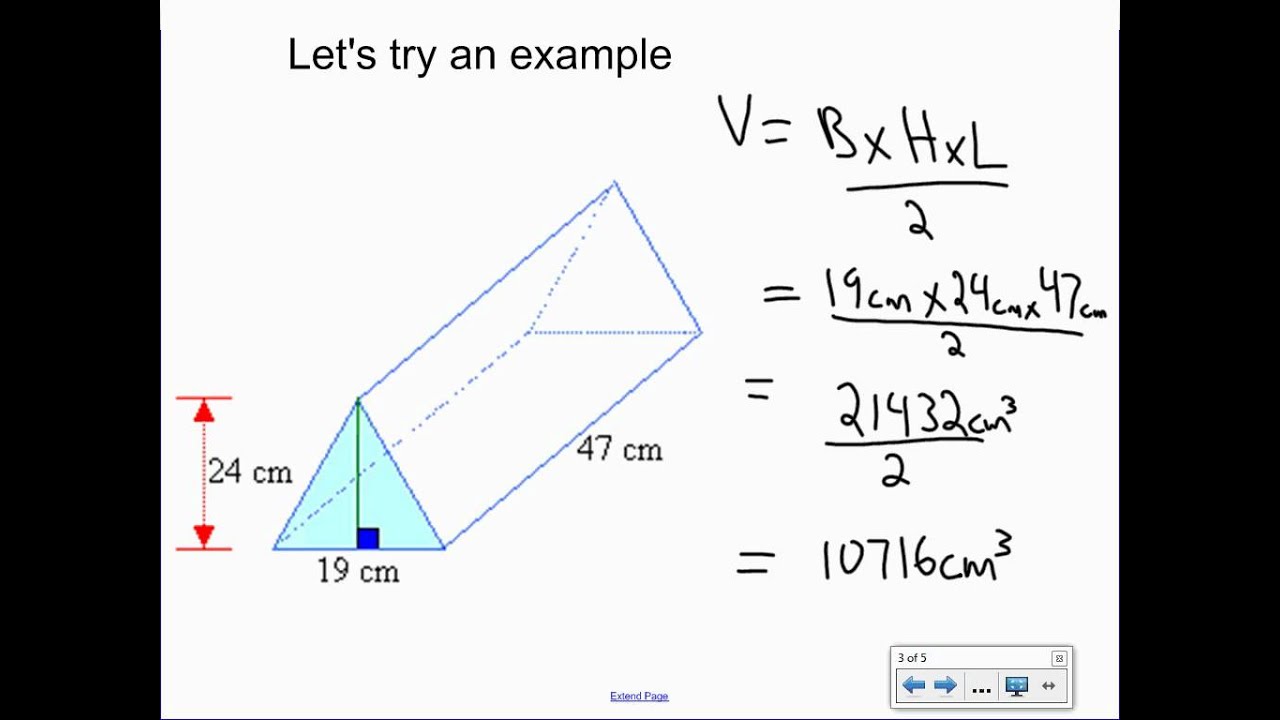
Since it is an area, surface area is measured in square units (e.g. The triangular faces of a triangular prism are congruent (exactly the same) but, unless the triangle is an isosceles triangle or an equilateral triangle, the rectangles are all different. The lateral surface area is the total area of the rectangular sides When finding the surface area of a triangular prism, we will often need to use Pythagoras ' theorem to find the length of one of the sides, or the height. For a triangular prism the top and the base are triangles and the lateral faces are rectangular sides. Lateral faces are all of the faces of an object excluding the top and the base. To work out the surface area of a triangular prism, we need to work out the area of each face and add them all together. Volume of a pentagonal prism = (0.3) (5) (0.The surface area of a triangular prism is the total area of all of the faces. NOTE: This formula is only applied where the base or the cross-section of a prism is a regular polygon.įind the volume of a pentagonal prism with a height of 0.3 m and a side length of 0.1 m. S = side length of the extruded regular polygon. The volume of a hexagonal prism is given by:Ĭalculate the volume of a hexagonal prism with the apothem as 5 m, base length as 12 m, and height as 6 m.Īlternatively, if the apothem of a prism is not known, then the volume of any prism is calculated as follows Therefore, the apothem of the prism is 10.4 cmįor a pentagonal prism, the volume is given by the formula:įind the volume of a pentagonal prism whose apothem is 10 cm, the base length is 20 cm and height, is 16 cm.Ī hexagonal prism has a hexagon as the base or cross-section. The apothem of a triangle is the height of a triangle.įind the volume of a triangular prism whose apothem is 12 cm, the base length is 16 cm and height, is 25 cm.įind the volume of a prism whose height is 10 cm, and the cross-section is an equilateral triangle of side length 12 cm.įind the apothem of the triangular prism. If the table-top really is supposed to be flat. The polygon’s apothem is the line connecting the polygon center to the midpoint of one of the polygon’s sides. If we allow the table-top to have one or more creases, then OP can subdivide the square prism into triangular ones and use the formula V 1 3A(a + b + c) where A is the volume of the triangular base, and a, b, c are depths to each vertex of the base. The formula for the volume of a triangular prism is given as Volume of a triangular prismĪ triangular prism is a prism whose cross-section is a triangle. Let’s discuss the volume of different types of prisms. Where Base is the shape of a polygon that is extruded to form a prism. The volume of a Prism = Base Area × Length The general formula for the volume of a prism is given as Since we already know the formula for calculating the area of polygons, finding the volume of a prism is as easy as pie. The formula for calculating the volume of a prism depends on the cross-section or base of a prism. The volume of a prism is also measured in cubic units, i.e., cubic meters, cubic centimeters, etc.
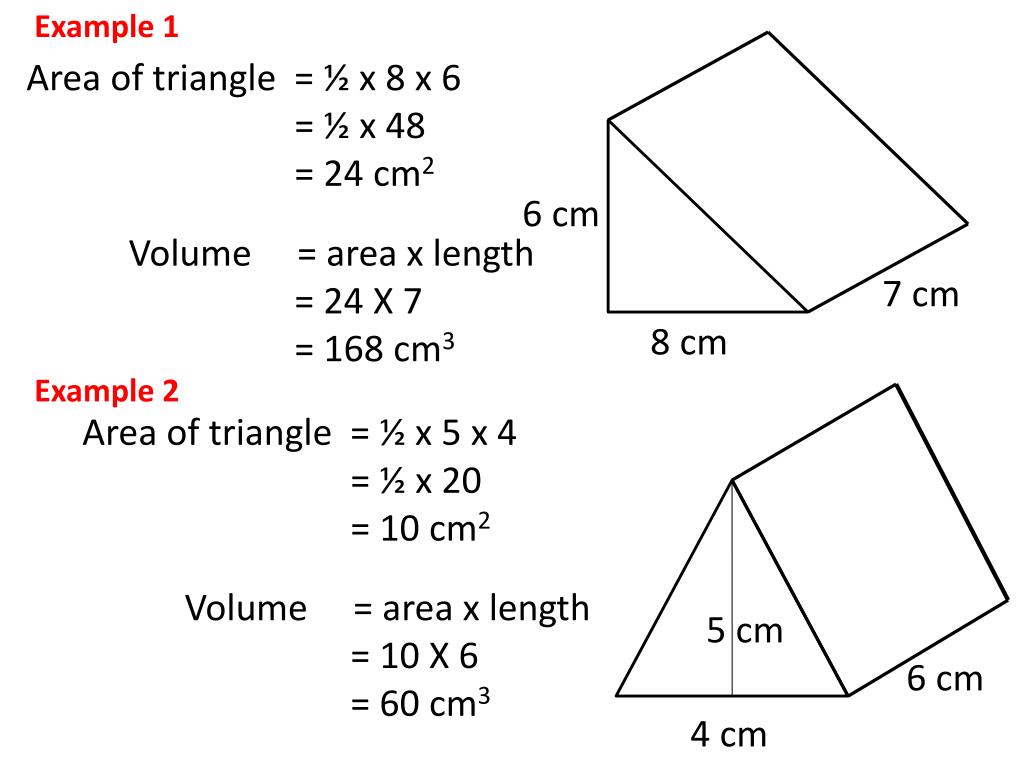
The volume of a prism is calculated by multiplying the base area and the height. To find the volume of a prism, you require the area and the height of a prism. pentagonal prism, hexagonal prism, trapezoidal prism etc. Other examples of prisms include rectangular prism. For example, a prism with a triangular cross-section is known as a triangular prism. Prisms are named after the shapes of their cross-section. By definition, a prism is a geometric solid figure with two identical ends, flat faces, and the same cross-section all along its length.
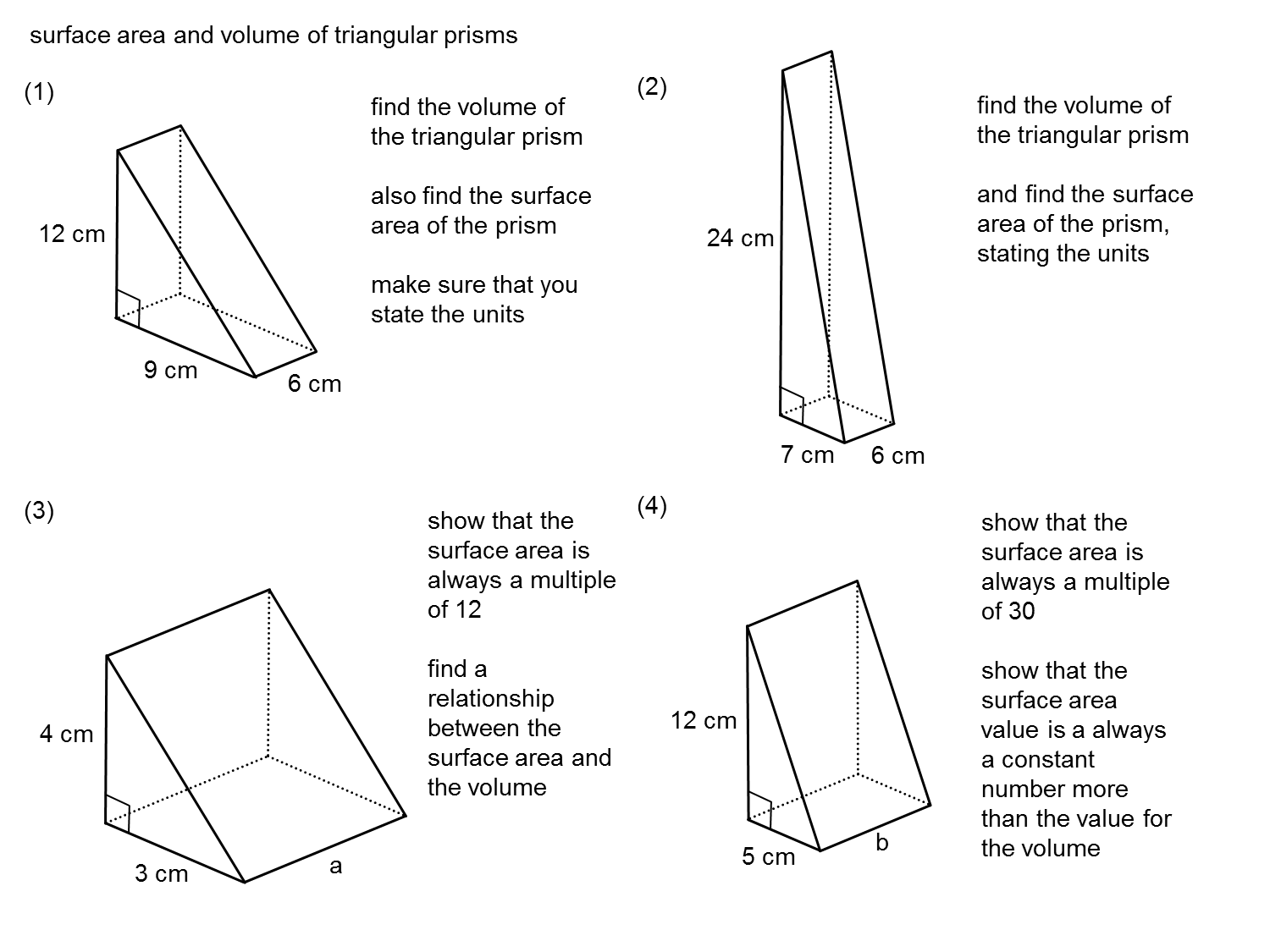
Finding the volume of triangular prism how to#
In this article, you will learn how to find a prism volume by using the volume of a prism formula.īefore we get started, let’s first discuss what a prism is. The volume of a prism is the total space occupied by a prism. Volume of Prisms – Explanation & Examples


 0 kommentar(er)
0 kommentar(er)
